how to find the constant of proportionality

Constant of Proportionality

When two variables are directly or indirectly proportional to each other, then their relationship can be described as y = kx or y = k/x, where k determines how the two variables are related to one another. This k is known as the constant of proportionality.

1.	What is Constant of Proportionality?
2.	Why Do We Use The Constant of Proportionality?
3.	How to Solve The Constant of Proportionality?
4.	Identifying The Constant of Proportionality
5.	Solved Examples
6.	Practice Questions
7.	FAQs on The Constant of Proportionality

What is Constant of Proportionality?

Constant of proportionality is the constant value of the ratio between two proportional quantities. Two varying quantities are said to be in a relation of proportionality when, either their ratio or their product yields a constant. The value of the constant of proportionality depends on the type of proportion between the two given quantities: Direct Variation and Inverse Variation.

Direct Variation: The equation for direct proportionality is y = kx, which shows as x increases, y also increases at the same rate. Example: The cost per item(y) is directly proportional to the number of items(x) purchased, expressed as y ∝ x
Inverse Variation: The equation for the indirect proportionality is y = k/x, which shows that as y increases, x decreases and vice-versa. Example:The speed of a moving vehicle (y) inversely varies as the time taken (x) to cover a certain distance, expressed as y ∝ 1/x

In both the cases, k is constant. The value of this constant is called the coefficient of proportionality. The constant of proportionality is also known as unit rate.

constant of proportionality in direct and inverse variation

Why Do We Use The Constant of Proportionality?

We use constant of proportionality in mathematics to calculate the rate of change and at the same time determine if it is direct variation or inverse variation that we are dealing with. Let us assume that the cost of 2 apples = $20. We determine that the cost of 1 apple = $10. We have found the Constant of Proportionality for the cost of an apple is 2.

If we want to draw a picture of the Taj Mahal by sitting in front of it on a piece of paper by looking at the real image in front of us, we should maintain a proportional relationship between the measures of length, height, and width of the building. We need to identify the constant of proportionality to get the desired outcome. Based on this, we can draw the monument with proportional measurements. For instance, if the height of the dome is 2 meters then in our drawing we can represent the same dome with height 2 inches. Similarly, we can draw other parts. In such scenarios, we use constant of proportionality.

Working with proportional relationships allows one to solve many real-life problems such as:

Adjusting a recipe's ratio of ingredients
Quantifying chance like finding odds and probability of events
Scaling a diagram for drafting and architectural uses
Finding percent increase or percent decrease for price mark-ups
Discounts on products based on unit rate

How to Solve The Constant of Proportionality?

We apply our knowledge on the direct and inverse variations, identify them and then determine the constant of proportionality and thereby get the solutions to our problems.

Example 1:Find the constant of proportionality, if y=24 and x=3 and y ∝ x.

Solution: We know that y varies proportionally with x. We can write the equation of the proportional relationship as y = kx. Substitute the given x and y values, and solve for k.

24 = k (3)

k = 24 ÷ 3 = 8

Therefore, the constant of proportionality is 8.

Example 2: 4 workers take 3 hours to finish the desired work. If 2 more workers are hired, in how much time will they complete the work?

Solution: Let x1 = number of workers in case 1 = 4

x2 = Number of workers in case 2 = 6

y1 = number of hours in case 1 = 3

y2 = number of hours in case 2 = To be found

If the number of workers is increased, the time taken to complete will reduce. We find that number of workers is inversely proportional to the time taken, (y1 = k/x1) ⇒ 3 = k / 4⇒ k = 12

Again, to find the number of hours, (y2 = k/x2) ⇒ y2 = 12/6= 2 hours.

Identifying The Constant of Proportionality

We shall now learn how to identify the constant of proportionality (unit rate) in tables or graphs. Examine the table below and determine if the relationship is proportional and find the constant of proportionality.

Number of Days = x	1	3	5	6
Number of Articles Written = y	3	9	15	18

We infer that as the number of days increases, the ariticles written also increases. Here we identify that it is in direct proportion. We apply the equation y= kx. To find the constant of proportionality we determine the ratio between the number of articles and the number of days. We need to evaluate for k = y/x

y/x = 3/1 = 9/3 = 15/5 = 18/6 = 3

From the result of the ratios of y and x for the given values, we can observe that the same value is obtained for all the instances. TheConstant of Proportionality is 3.

If we plot the values from the above table onto a graph, we observe that the straight line that passes through the origin shows a proportional relationship. The constant of proportionality under the direct proportion condition is the slope of the line when plotted for two proportional constants x and y on a graph.

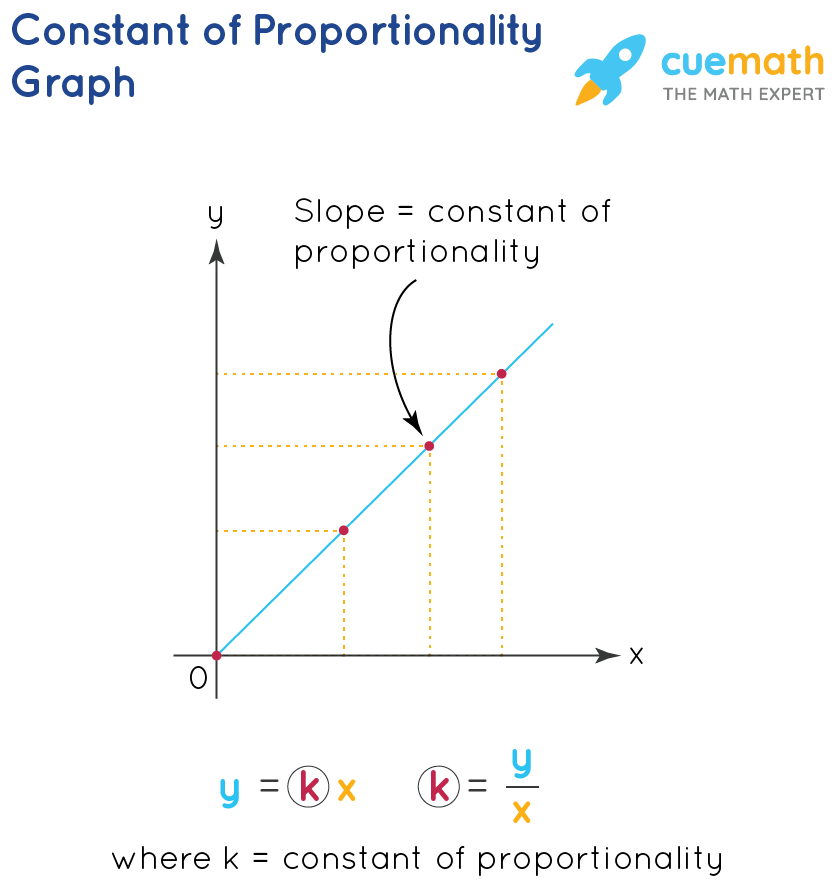
constant of proportionality in direct and inverse variation

Related topics to Constant Of Proportionality

Inverse Proportion Formula
Direct Proportion Formula
Constant of proportionality Calculator

Important notes

To check if the 2 quantities are proportional or not, we have to find the ratio of the two quantities for all the given values. If their ratios are equal, then they exhibit a proportional relationship. If all the ratios are not equal, then the relation between them is not proportional.
If two quantities are proportional to one another, the relationship between them can be defined byy = kx, where k is the constant ratio of y-values to corresponding x-values.
The same relationship can also be defined by the formula x=(1/k)y, where 1/k is now the constant ratio of x-values to y-values.

Example 1: Look at the table below. Do the variables exhibit any type of proportion? If so, what is the constant of proportionality?

X	Y
5	1
25	5
16	3
35	7

Solution:

To check the constant of proportionality, we use: y = kx

k = y/x

y/x = 1/5 = 5/25 =7/3 ≠ 3/16

We can observe that all the ratios in the above table are not equal. Hence, these values are NOT in a proportional relationship.

Example 2: Let us assume that y varies directly with x, and y=30 when x=6. Use the constant of proportionality and find the value of y when x=100.

Solution: Firstly, we should write the equation of the constant of proportionality, y = kx. Substitute the given x and y values, and solve for k. 30 = k × 6 ⇒ k=5

The equation is: y = 5x. Now, substitute x=100 and find y

y= 5 × 100 = 500
Therefore, the value of y is 500 when x=100.
Example 3: Anthony takes 15 days to reduce 30 kilograms of his weight by doing 30 minutes of exercise per day. If he exercises for 1 hour and 30 minutes every day, how many days will he take to reduce the same weight?

Solution:

According to the situation, weight and exercise are inversely proportional. As the number of minutes of workout increases, Anthony's weight reduces. Let m be minutes and d be days.
m =k/d. Let us have m1 = 30, m2= 90, d1 = 15. We are required to find d2.

m1 = k/d1
k = 30 × 15 = 450 (Substituting the known values, determine k)

m2 = k/d2

90 = 450/d2

d2 = 450/90 = 5(Substituting the known values, determine d2)

Therefore, if Anthony exercises for 1 hour and 30 minutes per day, it will take only 5 days to reduce 30 kilograms of weight.

go to slidego to slidego to slide

How can your child master math concepts?

Math mastery comes with practice and understanding the 'Why' behind the 'What.' Experience the Cuemath difference.

Book a Free Trial Class

FAQs on Constant of Proportionality

What is the Other Name for the Constant of Proportionality?

Another name for the constant of proportionality in mathematics is the unit rate.

What is the Constant of Proportionality in a Graph?

The straight line that passes through the origin is the constant of proportionality in a graph.

Why do we Use Constant of Proportionality?

We use constant of proportionality in mathematics to determine the nature of proportionality, whether it is direct proportion or indirect proportion. The constant of proportionality helps in solving the equations involving ratios and proportions.

What is the Constant of Proportionality of 12/6?

To find the constant of proportionality, in the case of direct proportionality, we use k=y/x. Let us take y = 12 and x = 6, then k = 12/6 = 2.

What is the Constant of Proportionality?

If the ratio of one variable to the other is constant, then the two variables have a proportional relationship, If x and y have a proportional relationship, the constant of proportionality is the ratio of y to x. Sometimes, we also represent it as x is to y.